Resumen
In this note we evaluate the unidimensional distributional Hankel transform of
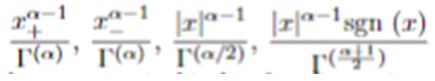
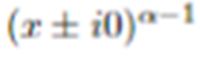
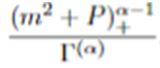
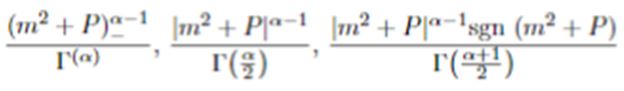
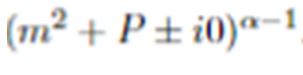
Citas
Schwartz, L. (1966) Théorie des distributions. Hermann, Paris.
Leray, J. (1952) Hyperbolic Differential Equations. Mimeographed Lecture Notes, Inst. for Advanced Studies, Princeton.
Gelfand, I.M.; Shilov, G.E. (1964) Generalized Functions. Vol I, Academic Press, New York.
Bresters, D.W. (1968) “On distributions connected with quadratic forms”, SIAM J. Appl. Math. 16: 563–581.
Aguirre, M.A.; Trione, S.E. “The distributional Hankel transform of δ(k)(m2+P)”, Studies in Applied Mathematics 83: 111–121, Massachusetts Institute of Technology Publ., Elsevier Science Publ. Co. Inc.
Trione, S.E. (1980) Distributional Products. Cursos de Matemática, N° 3, Serie II, IAM-CONICET.
Trione, S.E. (1987) “The multiplicative product between Pf(m2+P) and δ(γ−1)(m2+P), and others”, Trab. de Matemática, Serie I, N° 130, IAM-CONICET.
Bateman Manuscript (1953) Higher Trascendental Functions, Vol. I and II. McGraw-Hill, New York.
Grandshtegn, I.S.; Ryzhik, I.M. Table of Integrals Series and Products. Corrected and enlarged edition. Academic Press Inc.
Comentarios

Esta obra está bajo una licencia internacional Creative Commons Atribución-NoComercial-CompartirIgual 4.0.
Derechos de autor 1997 Manuel A. Aguirre





